Conference chapter: 'Shaping space: facial asymmetries in fifth-century Greek sculpture', Helle Hochscheid and Ronald Hamel

This chapter is intended to be read alongside and to complement the collection of images and essays on the Art of Making in Antiquity website. It results from conferences organised by the project in 2012 and 2013.

A downloadable PDF version of this essay (version 1.0) is available here. To cite this esssay please refer to this PDF version.

 

Abstract

The phenomenon of optical correction in Classical Greek sculpture has been attested by both ancient authors and modern scholars. Despite the apparent normalcy of optical correction in sculpture, however, there are no obvious reasons for it and how such correction worked in statues is a question which has rarely been examined systematically. Among the possible explanations are conscious application of philosophical or optical theories by sculptors, or subconscious influence of human visual perception on the carving process.

In this study, asymmetries (as one possible method of achieving optical correction) in the heads of extant fifth-century Greek sculpture have been analysed in relation to the ideal view and the pose of statues. All heads that were measured were asymmetrical, but statistical analysis showed no systematic pattern in the data that could be considered representative for sculpture from Classical Greece in general.

The lack of a statistically significant pattern means that (subconscious) correction of visual perception is not at the root of the asymmetries, undermining the notion of systematic application of contemporary optical theory by sculptors or the influence of their own visual perception. However, a practical explanation for the unsystematic asymmetries presented itself through interviews with seven sculptors, who pointed out technical reasons why asymmetries would occur naturally during carving, without influence of theoretical designs or visual perception.

Introduction

Visitor: One type of imitation I see is the art of likeness-making (εἰκαστικὴν τέχνην). That’s the one we have whenever someone produces an imitation by keeping to the proportions of length, breadth, and depth of his model, and also keeping to the appropriate colors of its parts.
Theaetetus: But don’t all imitators try to do that?
Visitor: Not the ones who sculpt or draw very large works. If they reproduced the true proportions of their beautiful subjects, you see, the upper parts would seem smaller than they should, and the lower parts would appear larger, because we see the upper parts from farther away and the lower parts from closer.
Theaetetus: Of course.
Visitor: So don’t those craftsmen say goodbye to truth, and produce in their images the proportions that seem to be beautiful instead of the real ones?
Theaetetus: Absolutely.

Plato Sophist 235d-236a

Rarely in ancient Greek literature does an author refer to the visual arts with such attention to the process of creation as Plato does in this quote from the Sophist.[1] Contrary to the more numerous considerations of philosophical aspects of artistic production in his work, for once Plato refers to the practical side of making sculpture: the correction of the optical distortion which occurs in the upper sections of statues that are considerably larger than life. That which distance makes appear too small to the viewer, he claims, artists correct by adjusting the proportions.

Theaetetus’ reaction seems to imply that optical correction was perfectly normal among the sculptors of Plato’s day, and even something that contemporary viewers might notice if omitted.[2] However, when looking at real people from an uncommon viewpoint, for example with strong foreshortening, human perception is used to compensating for the distorting effects of angle and distance.[3] So, when the human eye perceives a sculpted figure that looks like an over life-sized human being, why would this be any different? In other words, why would such optical adjustment be even necessary, let alone widespread?

Questions of asymmetry in scholarship

In the past, scholars have considered the presence of facial asymmetries a key indicator for optical correction in the heads of Greek sculpted figures.[4] One of the first scholars to relate facial asymmetries to optical correction was J. J. Winckelmann, who noted that in many statues, among others the famous Dying Niobid (cat. no. 39; fig. 1), the half of the face turned away from the viewer was flatter.[5] Since then, asymmetries have been observed in many individual statues; explanations for their occurrence range from aesthetic enhancement of the figure to technical errors by the sculptors.[6] Considering the high technical quality of much surviving classical sculpture, the latter seems an unlikely cause.[7] For example, a figure like the Herakles from the temple of Aphaia on Aegina (cat. no. 17; fig. 2) shows subtle but clear differences between the two halves of the face.[8] The position of this statue in the pediment would obscure these asymmetries, but during the carving process, they were clearly visible to the sculptor. The question is whether he carved the statue in this way purposely, and if so, what that purpose was.

Figure 1: Niobid, original in Rome, Museo delle Terme 72274. Plaster copy in the Abgussammlung Munich no. 1664, Cat. I, no. 40.
Image source: Schneider 1975: pl. 10. Reproduced by courtesy of Frank Steiner Verlag.

Asymmetry in sculpted figures could be attributed to deliberate effort, for example to achieve an effect of strong emotions (in later sculptural styles) or anatomical realism. Two studies from the 1970s by A. Stewart and I. McManus studied the patterns in the asymmetries of male genitals in Greek sculpture.[9] In more recent years, the latter author has shown that the statistical propensity for men's right testicles to be heavier and hang higher than the left ones is not reflected accurately in male statues from ancient Greece.[10] In many ways this outcome is not surprising, as fifth-century sculptors did not aim for realism. The idealised form of Classical sculpture would hypothetically preclude asymmetries, which would make them look notably less harmonious. Even though people's faces and bodies are invariably asymmetrical, the idealised beauty of Classical statues would have little to gain from asymmetry. So the question remains why the apparently flawless faces of fifth-century sculpture are nonetheless asymmetrical.

Figure 2: Herakles, East pediment of the Temple of Aphaia. Munich, Glyptothek, Cat. I, no. 17.
Image source: Schneider 1975: pl. 11. Reproduced by courtesy of Frank Steiner Verlag.

Before this can be explored further, it is important to distinguish between two kinds of facial asymmetries. On the one hand, asymmetries in the face and neck can result from movement, like they would in a real person. For example, a turn of the head to the proper (that is, the statue's own)  left lets the sterno-mastoid muscle on the right side of the neck stand out, changing the shape of the muscles around the jawbone. Equestrian figures often display an energetic turn of the head that results in such motion-related asymmetry, which may help to identify carved heads as belonging to horsemen.[11]

By contrast, structural asymmetries are beyond renderings of tendons and muscles in motion. The mouth or nose might be off-centre; the cheekbones might differ in width, height or volume; the eyes might be different sizes, etc. These asymmetries are not self-explanatory in high-quality idealising sculpture, since differently sized eyes or a crooked nose are, from a Classical point of view, less beautiful. An explanation like Plato’s optical correction for the latter type would be welcome: if asymmetries serve to increase the beauty when seen from one particular angle, the resulting lack of elegance from other sides could be justified by the improved effect when looking at the statue from the ideal viewpoint. An example of this is the pedimental figure of Theseus from the temple of Apollo in Eretria (cat. no. 46), whose features look, as Boardman observes, markedly better from below.[12]

If subtle differences between the two halves of the face work in this way to enhance the effect of the statue from one viewpoint, it becomes possible to deduce this ideal viewpoint by observing from which side it works best aesthetically.[13] The principle of creating an ideal viewpoint through asymmetries is often used for the reconstruction of statues: not only can its pose be established based on muscular or motion asymmetries, but structural asymmetries can help identify the original intended angle for viewing a head, particularly when the corresponding body or original placement on a pedestal are no longer preserved.[14] In this way, asymmetries can be helpful for example in the reconstruction of sculptural groups.

In order for an ideal viewpoint to function in this way, the asymmetries which create it must follow a recognisable pattern. Theoretically, optical correction serves to counter distorting effects of our visual perception, or put positively, to enhance the visual experience of a statue at least from one angle, by taking advantage of how our visual perception functions. However, for this ideal view to be recognisably more pleasing, viewers must agree on what constitutes the statue’s ‘good side’, whether they do so instinctively or based on scholarly arguments. If, therefore, asymmetries contribute to the ideal viewpoints of statues, they must follow a pattern that a majority of viewers would acknowledge consciously or subconsciously as effective.

Thus, an investigation of the possible explanations for asymmetries must start with establishing whether a pattern does indeed exist. This raises immediate problems. First, original Classical Greek sculpture survives in limited numbers, and when it does its condition is often quite poor. Second, if a pattern could be found, the explanation of purposeful optical correction requires a level of theoretical awareness among ancient sculptors that can is questionable. A connection between sculptors and philosophers such as Plato is not sine qua non established, and there is a possibility that Plato’s notion of correction had little to do with the practical reality of sculpture in the fifth and fourth centuries BC. Although the scope of this article does not warrant a full discussion of this subject, some brief remarks are in order.

Literary evidence for awareness of anatomy, complex geometry or optical theories among sculptors of the fifth century BC is scanty.[15] The accuracy of anatomical detail in Classical sculpture has led some scholars to postulate medical knowledge among them. [16] Complex geometry was applied famously in fifth-century architecture, and treatises about this are known to have been written from the early archaic period onward.[17] In sculpture, Polykleitos was famous for having put down in writing a system of proportional measurements for his statues.[18] These examples suggest that knowledge of geometry and certainly of the proportions of the human body was not uncommon among sculptors of the fifth century. In view of the quality of the surviving sculpture, it does not seem too much of a leap to think them capable of guiding their viewers’ glances. But ultimately, contemporary literary sources that aim to present the practices of sculptural production, or even the perceptions of sculpture by the public, are missing. The only direct source available to modern scholars are preserved Classical statues. Despite their limited numbers, these need to be investigated to see whether the optical correction from ancient texts can be related to the evidence of sculpture.

Asymmetries in sculpted faces: theories, methods, data

Whether asymmetries are the result of subconscious influence of human visual perception on the carving process, or of conscious distortion to enhance the aesthetic effect of a finished statue, it is essential to determine whether facial asymmetries in Greek sculpture show any significant regularity.[19] Based on the surviving corpus of classical sculpture, facial asymmetries in Greek sculpture can, and have been, examined. The ‘rule’ for the ideal viewpoint (also referred to as the viewpoint theory) is usually that the half of the face that is turned towards the viewer appears larger and flatter than the turned-away half.[20] In compensation, the latter is made longer and wider, and the front half more strongly curved.

Few studies, however, have systematically investigated the principles behind this. Descriptions which are detailed enough to include asymmetries are confined to a few heads, making it impossible to establish whether there is a more universal pattern. An exception is Lambert Schneider’s 1975 work Asymmetrie griechischer Köpfe. He argued that not the ideal viewpoint determines the asymmetries, but the movement of the statue’s head. For example, the head of the Zeus of Cape Artemision is turned vigorously to the proper left, but, Schneider noted, the left half of the face is not taller than the right half, as in the viewpoint theory.[21] Rather, the vertical and horizontal axes of the face are bent as if swept up by the movement of the head, converging in this case to the proper left (fig. 3, 1–3). A similar convergence occurs in the head as a whole: the line which runs from the middle of the forehead to the middle of the back of the head bends to form a concave line towards the proper left (fig. 3, 4–5). This causes the turned-away half of the face to be lower and narrower, the effect increasing towards the outside of the face. Schneider called this motion theory, and tested it on 319 heads from Classical and Hellenistic sculpture. He presented the results in tabulated form, using symbols to indicate whether his pattern was confirmed or negated by the evidence. In a majority of cases his data supported the theory, and some considered the discussion on facial asymmetries closed.[22]

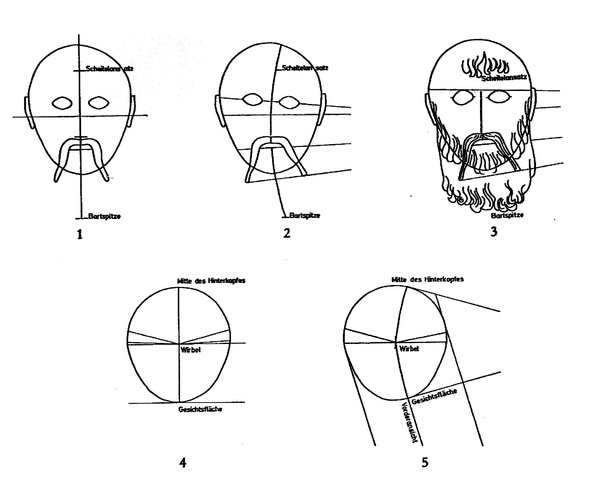
Figure 3: Schematic overview of motion distortion in the Zeus of Cape Artemision.
Image source: Schneider 1975: 12–14. Reproduced by courtesy of Frank Steiner Verlag.

However, neither Schneider nor adherents of the viewpoint theory take into account the methodological problem of the fact that not all Greek sculpture from the fifth century is preserved. A pattern in the asymmetries in any collection of surviving sculpture might be completely coincidental. So, after establishing the existence of a pattern, a further step must be to test whether it would hold up if one could perform the same test on the hypothetical body of all Greek sculpture.[23] With the help of statistics, the chance can be calculated that the asymmetries in the surviving fifth-century sculpture are likely to have been a universal phenomenon, or are just a coincidence in the surviving material.

The statues in the catalogue are treated as a random sample rather than as a full population (which would be all Greek sculpture produced in the fifth century). If the hypothesis of optical correction, either along the lines of movement or of viewpoint, appears in a significant number of statues and according to a regular pattern, the use of optical correction through asymmetries can be considered a general characteristic of Greek sculpture from this period, and it becomes fairly certain that sculptors applied these asymmetries purposely and consciously.

In the descriptive catalogue, eighty-two sculptures are listed, their dates ranging from the late archaic period to the end of the fifth century BC, with an additional seven Roman copies of classical statues (Catalogue 1, available at https://roac.academia.edu/HelleHochscheid).[24] More than half of these are architectural figures: for pedimental figures and high-relief metopes, original poses and positions of the figures are often known, and so the necessary information on poses and possible viewing angles of the figures is more likely to be available.[25] Among the sculpture studied here are figures from Temple E in Selinunte, the Temple of Apollo in Eretria, the Aphaia Temple on Aegina, the Temple of Zeus in Olympia, the Parthenon, and the pediments of a Greek temple which were later used in the Temple of Apollo Sosianus in Rome. Some heads of freestanding single statues from the fifth century are also part of the evidence.[26] The measurements on the pediments of the Aphaia Temple on Aegina were taken from the originals, all others from plaster casts in the collections of the universities of Bonn and Munich.[27]

Measurements were taken from ten points on each head, providing information for both halves of the faces, and assessed with the naked eye in a further twelve (Catalogue 2, available at https://roac.academia.edu/HelleHochscheid).[28] An example of the results, the measurements of figure A from the West pediment of the Zeus temple in Olympia, is presented in the Table below. These data were first standardised, and then compared with the demands of the motion theory and the theory of an ideal viewpoint. The standardisation of each difference was achieved by submitting the value to this formula:

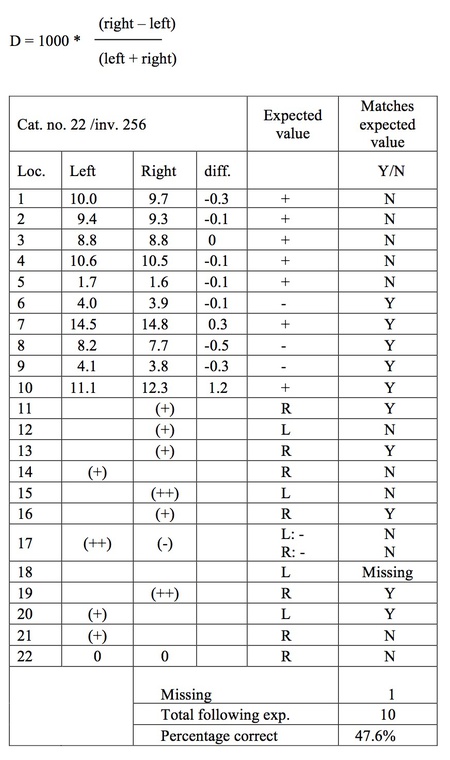
Example of measured asymmetries in twenty-two locations and expected values based on the statue's pose

The left-hand column indicates the points of measurement on the head, with the first ten locations represented in numbers, the final twelve in larger/more strongly curved (+) or equally large/equally strongly curved. The fifth column indicates what for a hypothetical ideal viewpoint or for the movement in this statue the asymmetry should be; the final column describes whether or not this expectations is met.

In this way, one standardised number, rounded to one decimal, was obtained for each measuring location of each statue (Catalogue 2, Sheet 2), resulting in ten numerical data per statue, or fewer in case of damage. The next step was to list the statues according to ideal view or motion. Both categories have three possible subgroups, namely frontal, left, and right (either in profile or in three-quarter view or position). Within each of the six subgroups, the results for all measuring locations have been submitted to a t-test (Tables 3–4). This test calculates the chance that one would find the results as they are in the dataset, if all the sculpted heads which once existed in fifth-century Greece had, on average, had a difference of zero between the left and right halves of the faces. The standardised results of the measurements show clear differences between the left and right halves of a considerable number of the statues in the database. The t-test investigates whether this is more likely to be a coincidence of the surviving material, or whether it is sufficiently representative to assume that all sculpture had such differences.[29] The threshold p used for this chance was set at 5% (p = 0.05): if the chance of a coincidence was larger than 5%, one could reject the theory that asymmetries were a systematic phenomenon in Greek sculpture.[30]

Only two of the fifty asymmetries (D8 for statues with movement to the proper right, see Table 3a, and D10 for statues with a frontal position, see Table 3c) are significant. The results are clear: although the statues all have visible and measurable asymmetries, the chance that this is coincidental is too large to assume a universal use in Greek sculpture of the fifth century BC.[31]

The t-test poses strict criteria to not only the direction of the asymmetries, but also to their size. The latter aspect is less relevant in the present case, since the difference between the distances in the right and left half of the heads are often quite small. It is in fact more important whether the standardised value is positive or negative, and whether this is in accordance with the expectancy of either ideal viewpoints or motion theory. These questions can be examined by means of a sign test (Tables 5–7). The population median was set at zero, and the numerical data were used to see whether the differences between left and right, regardless of their size, result in the expected negative or positive direction, or whether they cancel each other out (Tables 5a–b). Again, a threshold is set in order to differentiate between a chance and a systematic phenomenon: the values have to fall outside of a preset margin around the population median. If the results were to fall within this margin, the probability is too large and the zero hypothesis is assumed to be correct. In other words, the population median set at zero represents the hypothesis that on average, the differences between left and right cancel each other out, and if the results of the sign test approach this zero hypothesis too much, it becomes more likely that the differences in the directions of the asymmetries in the database are coincidental.

This test, too, was applied for both the ideal viewpoint and the motion theories (i.e. related to the rule of correction for a better aesthetic effect in the former, and the enhancement of the movement by bending the axes according to the latter). Again the outcomes were non-significant, and so it must be concluded that the asymmetries in the dataset do not represent a universal pattern of systematic asymmetries in fifth-century Greek sculpture.[32]

The conclusion is that the asymmetries in the faces of sculpture are not a systematic phenomenon. The first implication of this outcome is that it is problematic to use structural facial asymmetries for reconstructing an ideal viewpoint, and by extension, for reconstructing poses of statues whose bodies are not preserved or the composition of pediments. Second, the evidence does not confirm the underlying assumption of deliberate optical corrections in sculpture. Evidently sculptors applied no systematic alterations to the proportions of the heads of their statues, and were not inclined to theoretical rigour in statues' proportions or optical correction.[33] Consequently, there is no confirmation here of literary testimonia that claim philosophical or optical education of sculptors or their acceptance in intellectual circles in fifth-century Greece.[34] However, non-significant statistic results like those presented here often suggest multiple causes of the phenomenon that is being examined. Even if asymmetries in sculpture are non-systematic, their presence still needs to be explained.

Answers from the workshop

Even though Plato’s assertions of purpose and system in the optical corrections in sculpture cannot be accepted for the extant material, an explanation is still due for the omnipresent, if random asymmetries in the heads and faces of the classical Greek statues in this study. With this in mind, seven sculptors were interviewed.[35] The questions focused on the carving of figurative statues rather than directly on asymmetries, in order to find out more about when and how in this creative process asymmetries might occur in the opinion of each sculptor.[36] Had the outcome of the quantitative research been positive, it would have stood to reason to expect Classical sculptors to plan asymmetries in advance, when the composition of the statue was designed.

The interviews confirmed what the statistics had suggested: none of the sculptors planned asymmetries or any form of optical correction in the early stages, when they were designing the statue in sketches on paper, in clay, or both. This could, of course, simply be a difference between ancient and contemporary sculptors. But the absence of a system in the asymmetries in ancient sculpture implies a similar lack of deliberate planning by fifth-century sculptors. The interviewees all confirmed that even if had they wanted to use asymmetry for optical purposes, they would not have planned for it early on, i.e. in the design stage. They proposed that the asymmetries would have appeared in the head of a statue relatively late in the carving process. In fact, they agreed on three very practical aspects of the carving that they considered highly conducive to asymmetries like those found in classical Greek sculpture.

The first relevant factor for a sculptor carving a statue would be the literal limits of his or her perception. Sophisticated optical illusions and distorting effects aside, every sculptor has a very basic problem: he or she cannot see two sides of a block of marble simultaneously. When working on the front of a statue, the impact of the work on the adjoining faces cannot in the same glance be observed. A remedy could be to use high-quality mirrors (which would be limited in antiquity) or to work round in planes: instead of cutting the statue out of the block from front to back, layers of material would then be removed all around the statue like the peel of an apple.[37] This is, however, only a partial solution, because once the final surface of the statue is almost reached and details such as the cheeks and eyes are taking shape, the same problem would occur, albeit on a subtler scale.[38] So, the inability of the human eye to see around a corner influences the symmetry of a statue, because without artificial help it is not possible to monitor continuously what consequences the work on one side of the statue has on the adjoining surfaces.

A second possible cause of asymmetries which the interviewed sculptors brought up, was their own laterality. A right-handed sculptor would hold the mallet with his right hand, and the chisel with his left hand. While carving the right side of the statue’s head, this would be a convenient situation; but as soon as she or he would move to its left side, the carving would become very awkward. A solution could be to switch hands, and although this is possible (even a very inexperienced sculptor like myself is able to do so), the control over the mallet is much less in one’s non-preferred hand. With training, the difference becomes smaller, as experience shows, but even so it is near-impossible to do precisely the same with both hands.

Alternatively, the problem could be remedied by standing on the different sides of the statue when carving different parts. In the example, this would be the statue’s left-hand or rear side, working from the back to the front. However, in terms of asymmetries this would potentially have even greater consequences: coming via the left side of the head to the front, it would be impossible to see the left half of the face, short of stepping forward to take a look. So, considering the impact of right or left-handedness on the carving, it would in fact be surprising if any statues had symmetrical faces, because there have to be either two different lines of sight while carving or one has to use different hands for different sides. The combination of visual perception, hand-eye coordination and laterality makes it virtually impossible to obtain identical results on two sides.

A third aspect is the structure of some types of marble. Several of the sculptors who were interviewed had experience with various marble types, and they singled out certain types from Paros or other Cycladic islands. Contrary to, for example, marble from Carrara, some of the Cycladic marbles have oblong crystals. This means that their molecules are set, not in more or less cubic structures, but in bar-shaped, rectangular ones. According to the interviewees, the effect of this crystalline structure of the marble on the carving process is similar to that of wood: when going along the grain, the resistance is much lower and the cutting much smoother than when going against it. In the case of marble, going against the grain entails breaking the crystals to go through them, while going along them means that the chisel can follow the longer side of the crystals at a much lower resistance, only breaking the marble between crystals. If rectangular-grained marble were used, its structure would have had consequences for the carving of details such as, for example, facial features.

Shaping space

When Plato commented on the use of false proportions to increase the aesthetic appeal of large statues, he hardly intended to lecture on the creation of sculpture. His point was about the value of numbers and measurements over mere visual perception. But ironically, exact measuring and quantitative analysis of fifth-century sculpture belie Plato’s criticism. Instead, the evidence suggests that sculptors in Classical Greece did not purposely change the proportions of their statues’ heads to enhance one viewpoint, nor to enhance its sense of motion. The fact that each statue in this study has proven to be asymmetrical has to be explained in other ways.

The suggestions offered here point to a combination of factors, among which are the laterality of sculptors, the inability of the human eye to observe more than one side of a statue simultaneously, and in specific cases, the crystalline structure of the marble that was used. All of these aspects originate from practical or technical circumstances occurring quite close to the completion of the statue, rather than from the execution of deliberate and meticulously planned design. It is this variety of practical causes, all related to involuntary processes during carving, that brings about the un-systematic phenomenon of asymmetries of the statues in evidence. The fact that scholars, and perhaps even Plato, thought to see deliberate patterns in these asymmetrical faces, is a tribute to the quality of these sculptors and their work.

Dr. Helle Hochscheid
University College Roosevelt (Utrecht University)
Email: h.hochscheid@ucr.nl

Dr. Ronald Hamel
University of Amsterdam
Email: r.hamel@uva.nl

References

Ahrens, D. (1983). Entwicklung und Nachleben antiker Meßsysteme in der Bildhauerkunst’, Jahreshefte des Österreichischen Archäologischen Institutes in Wien 54: 113–24.

Alscher, L. (1954-1982).Griechische Plastik. Berlin.

Ashmole, B. (1951). ‘Demeter of Cnidus’, Journal of Hellenic Studies 71: 13–28.

Balensiefen, L. (1994). ‘Die 'Kyropädie' des Baumeisters Kyros und die antiken Sehtheorien’, Jahrbuch des Deutschen Archäologischen Instituts 109: 301–19.

Berger, E., Müller-Huber, B., and Thommen, L. (1972). Der Entwurf des Künstlers Bildhauerkanon in der Antike und Neuzeit. Basel.

Borbein, A. (1996). ‘Polykleitos’ in O. Palagia and J. J. Pollitt (eds).Personal styles in Greek sculpture (Yale classical studies 30). Cambridge: 66­­–90.

Brouskari, M. (1974).The Acropolis Museum: a descriptive catalogue (Commercial Bank of Greece. Publications Series 2; The museums of Greece 1). Athens.

Brunnsåker, S. (1971).The Tyrant-Slayers of Kritios and Nesiotes: a critical study of the sources and restorations (Skrifter utgivna av Svenska institutet i Athen 17). Stockholm.

Cook, R. M. (1973). Review of B. Ashmole, Architect and Sculptor in Classical Greece. London.

Coulton, J. J. (1977). Greek architects at work. Ithaca NY.

De Lacy, Ph. ed. (1984). Galeni De placitis Hippocratis et Platonis, Corpus medicorum Graecorum, Vol. 4, Berlin.

Diels, H.A., E. Schramm eds. (1970). Herons Belopoiika (Schrift vom Geschützbau). Philons Belopoiika (Mechanik Buch IV und V), Vol. II. Leipzig.

Edwards, C. (1996). ‘Lysippos’, in O. Palagia and J. J. Pollitt (eds).Personal styles in Greek sculpture (Yale classical studies 30). Cambridge: 130–53.

Goldhill, S. and Osborne, R. (eds). (1994).Art and text in ancient Greek culture. Cambridge.

Hampe, R. (1960). ‘Rezension zu Fouilles de Delphes, Tome IV: Monuments figurées, Sculpture. Fasc. V: F. Chamoux, L'Aurige’, Gnomon 32: 60–73.

Helmreich, G. ed. (1969). Galeni De temperamentis libri III. Stuttgart.

Hurwit, J. (1989). ‘The Kritios Boy: discovery, reconstruction, and date’, American Journal of Archaeology 93: 41–80.

Hurwit, J. (1995). ‘The Doryphoros: looking backward’, in W. G. Moon (ed.). Polykleitos, the Doryphoros, and tradition. Madison WI: 3–18.

Knell, H. (1986). Symmetrie in der Antike’, in B. Krimmel (ed.). Symmetrie in Kunst, Natur und Wissenschaft. Darmstadt: 157–76.

Krimmel K. (ed.). (1986). Symmetrie in Kunst, Natur und Wissenschaft. Darmstadt.

Langlotz, E. (1951).‘Eine Artemiskopf’, in G. E. Mylonas and D. Raymond (eds). Studies presented to David Moore Robinson on his seventieth birthday, Vol. 1. Saint Louis MO: 638–47.

Leftwitch, G. (1995). ‘Polykleitos and Hippocratic medicine’, in W. G. Moon (ed.). Polykleitos, the Doryphoros, and tradition. Madison WI: 38–51.

Lippold, G. (1950). Die griechische Plastik (Handbuch der Archäologie im Rahmen des Handbuchs der Altertumswissenschaft 3).Munich.

Lepik-Kopaczynska, W. (1959). ‘Die optischen Proportionen in der antiken Kunst, Klio 37: 67–101.

Mark, I. (1995). ‘The lure of philosophy: craft and higher learning in ancient Greece’, in W. G. Moon (ed.). Polykleitos, the Doryphoros, and tradition. Madison WI: 25–37.

McManus, I. C. (2004). ‘Right-left and the scrotum in Greek sculpture’, Laterality 9: 189­–99.

McManus, I. C. (2005). ‘Symmetry and asymmetry in aesthetics and the arts’, European Review 13: 157–80.

Métraux,G. P. R. (1995). Sculptors and physicians in fifth-century Greece: a preliminary study. Montreal.

Mithen, S. (1996). The Prehistory of the Mind: The Cognitive Origins of Art, Religion and Science. London.

Osborne, R.  (1994). ‘Looking on – Greek style. Does the sculpted girl speak to women too?’, in I. Morris (ed.). Classical Greece: ancient histories and modern archaeologies. Cambridge: 81–96.

Osborne, R. (2000). ‘Archaic and Classical Greek temple sculpture and the viewer’, in N. K. Rutter and B. A. Sparkes (eds). Word and image in ancient Greece (Edinburgh Leventis studies 1). Edinburgh: 228–46.

Osborne,R.(2011). The history written on the classical Greek body. Cambridge.

Palagia,O. (1980). Euphranor (Monumenta Graeca et Romana 3). Leiden.

Palagia, O.(2008). Greek sculpture: function, materials, and techniques in the archaic and classical periods. Cambridge.

Pollitt, J. J. (1972).Art and experience in classical Greece, Cambridge.

Pollitt, J. J. (1974).The ancient view of Greek art: criticism, history, and terminology. New Haven, CT.

Pollitt, J. J. (1995). ‘The canon of Polykleitos and other canons’, in W. G. Moon (ed.). Polykleitos, the Doryphoros, and tradition. Madison, WI: 19–24.

Pollitt,J. J. (2001). The art of ancient Greece: sources and documents. 2^nd edition. Cambridge.

Porter,J. I.  (2010). The origins of aesthetic thought in ancient Greece: matter, sensation, and experience. Cambridge.

Richter,G. M. A. (1970). Perspective in Greek and Roman art. London.

Ridgway, B. S. (1976). ‘Review of Asymmetrie griechischer Köpfe vom 5. Jh. bis zum Hellenismus, by Lambert A. Schneider’, American Journal of Archaeology 80: 93–4.

Ridgway,B. S. (1993). The archaic style in Greek sculpture. 2^nd edition. Chicago IL.

Ridgway, B. S. (2001).Hellenistic Sculpture I: the styles of ca. 331-200 B.C. Madison WI.

Ridgway, B. S. (2000). Hellenistic Sculpture II: the styles of ca. 200-100 B.C. Madison WI.

Ridgway, B. S. (2002). Hellenistic Sculpture III: the styles of ca. 100- 31 B.C. Madison WI.

Ridgway, B. S. (2004). Second chance: Greek sculptural studies revisited. London.

Rouveret,A. (1989). Histoire et imaginaire de la peinture ancienne: V^e siècle av. J.-C.- I^er siècle ap. J.-C. (Bibliothèque des écoles françaises d'Athènes et de Rome 274). Rome.

Sacks, O. (2010). The mind's eye. London.

Schneider,L. (1973). Asymmetrie griechischer Köpfe vom 5. Jh. bis zum Hellenismus. Wiesbaden.

Schrader, H., Langlotz, E. and Schuchhardt, W. H. (1939).Die archaischen Marmorbildwerke der Akropolis. Frankfurt am Main.

Senseney,J. R. (2011). The art of building in the classical world: vision, craftsmanship, and linear perspective in Greek and Roman architecture. Cambridge.

Skoyles, J. R. (1998). ‘Motor perception and anatomical realism in Classical Greek art’, Medical Hypotheses 51: 69–70.

Steiner, D. (2001).Images in mind: statues in archaic and classical Greek literature and thought. Princeton NJ.

Stewart, A. F. (1976). ‘Scrotal asymmetry: an appendix’, Nature 262: 155.

Stewart, A. F. (1978). ‘The canon of Polykleitos: a question of evidence’, Journal of Hellenic Studies 98: 122–31.

Stewart,A. F. (1990). Greek sculpture: an exploration. New Haven CT.

Tanner, J. (2006).The invention of art history in Ancient Greece: religion, society and artistic rationalisation. Cambridge.

Tatarkiewicz, W. (2005).History of aesthetics. London.

Thibodeau, P. (2003). ‘Optical illusions in Ancient Greece’, in G. W. Bakewell and J. P. Sickinger (eds). Gestures: essays in ancient history, literature, and philosophy presented to Alan L. Boegehold on the occasion of his retirement and his seventy-fifth birthday. Oxford: 136–51.

Tobin, R. (1990). ‘Ancient perspective and Euclid's Optics’, Journal of the Warburg and Courtauld Institutes 53: 14–41.

Tobin, R. (1995). ‘The pose of the Doryphoros’, in W. G. Moon (ed.). Polykleitos, the Doryphoros, and tradition. Madison WI: 52–64.

Trianti,I. (1998). To Mouseio Akropoleos. Athens.

White. N. P. (1997). Sophist by Plato. Translation in J. Cooper (ed.) Plato. Complete Works. Indianapolis, IN.

Winckelmann, J. J. and Eiselein, J. (eds). (1965).Johan Winckelmanns sämtliche Werke: Einzige vollständige Ausgabe; dabei Porträt, Facsimile und ausführliche Biographie des Autors; unter dem Texte die frühern und viele neue Citate und Noten; die allerwärts gesammelten Briefe nach der Zeitordnung, Fragmente, Abbildungen und vierfacher Index. Osnabrück.

Zeki, S. (2001). ‘Artistic creativity and the brain’, Science 293, no. 5527: 51–2.

Zeki, S. (2005). ‘The Ferrier Lecture 1995 - Behind the Seen: The functional specialization of the brain in space and time’, Philosophical Transactions of the Royal Society-Biological Sciences 360: 1145–83.

Zinserling, G. (1976). Rezension zu Asymmetrie griechischer Köpfe vom 5. Jh. bis zum Hellenismus, Deutsche Literaturzeitung 97: 69–71.

Appendix: list of measuring points

Measuring points are partly derived from Schneider 1975, 8–10. The accuracy of the measurements was impeded by several factors: the callipers end not in a point but in a small flat surface, which made measuring safer for the surface of the statue, but less precise. Moreover, some statues were so small that the callipers would not fit in the corner of an eye or mouth. Smaller statues have mostly been avoided for this reason, but on the other hand, it seems that in the ones which were included, the asymmetries are relatively conspicuous.

1           Corner of the mouth – inner corner of the eye (measured on the outer corner, lower side of the upper lip / above of tear duct on the eyeball)
2           Corner of the mouth – outer corner of the eye (measured as in point 1 / top of the lower eyelid on the eyeball, outer corner of the eye)
3           Corner of the mouth – middle of the lower eyelid (measured as in point 1 / top of the lower eyelid on the eyeball)
4           Corner of the mouth – middle of the upper eyelid (measured as in point 1 / bottom of the upper eyelid on the eyeball)
5           Height of the eye (tallest part of the eye, measured on the eyeball)
6           Width of the eye (between the tear duct and the outer corner, measured on the eyeball)
7           Corner of the mouth – start of the earlobe (measured at the top of the jawbone, where the ear starts from the head; if this location was damaged, the lowest point of the antitragus)
8           Middle of the nose bridge – outer corner of the eye (measured at the top end of the nasal bones)
9           Corner of the mouth – middle of the mouth (measured on the location of the upper lip as in point 1 / in the middle of the philtrum)
10        Outer corner of the eye to the start of the earlobe (measured as in point 2 / as in point 7)

The measurements provided two numerical outcomes per location, one for each side of the face. However, since callipers rather than tape were used for measuring, equal values for both sides of the face might not coincide with similar surfaces. A stronger curve of the cheek on one side would not influence the width measured by callipers, but measuring tape would show a longer distance. More importantly, the effect would be very different from a gentler surface. In order to offer a complete picture of the asymmetries of each face, some more qualitative data was therefore recorded, describing non-quantified differences visible to the naked eye:

11        Circumference of the two halves of the head
12        Placement of the eyes relative to each other / the eyes’ axis
13        Nasal axis relative to eyes’ axis
14        Axis of the mouth relative to that of the nose
15        Mouth relative to nasal axis (i.e. whether the nose is placed symmetrically below the nose or off-axis)
16        Philtrum relative to nasal axis
17        Difference between inner and outer corner of each eye relative to eyes’ axis
18        Parting of the hair (if present and central) relative to the vertical central axis of the face
19        Hairline on two sides of the vertical central axis of the face (i.e. where more or less of the face is exposed)
20        Width of the two halves of the face, from frontal view
21        Curve of the cheeks, mostly horizontal but if applicable also vertical
22        Transition front to side of the head (i.e. a more or less square shape of the skull, or the angle of transition of the planes)

The numbers in which these measurements resulted and the statistical analysis performed on them is presented in Catalogue 2, the quantitative catalogue.